How To Difference Between Empty Set (Null Set) And Subsets:
So let’s go back to our definition of subsets. We have a set A. We won’t define it any more than that, it could be any set. Is the empty set a subset of A?
Going back to our definition of subsets, if every element in the empty set is also in A, then the empty set is a subset of A. But what if we have no elements?
It takes an introduction to logic to understand this, but this statement is one that is “vacuously” or “trivially” true.
A good way to think about it is: we can’t find any elements in the empty set that aren’t in A, so it must be that all elements in the empty set are in A.
So the answer to the posed question is a resounding yes.
The empty set is a subset of every set, including the empty set itself.
As an example, think of the set of piano keys on a guitar.
“But wait!” you say, “There are no piano keys on a guitar!”
And right you are. It is a set with no elements.
This is known as the Empty Set (or Null Set).There aren’t any elements in it. Not one. Zero.
“But wait!” you say, “There are no piano keys on a guitar!”
And right you are. It is a set with no elements.
This is known as the Empty Set (or Null Set).There aren’t any elements in it. Not one. Zero.
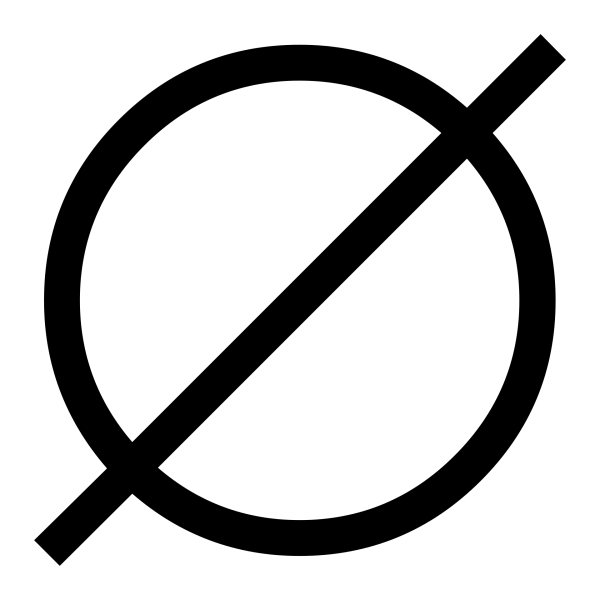
It is represented by
Or by {} (a set with no elements)
Some other examples of the empty set are the set of countries south of the south pole.
So what’s so weird about the empty set? Well, that part comes next.
Some other examples of the empty set are the set of countries south of the south pole.
So what’s so weird about the empty set? Well, that part comes next.

Comments
Post a Comment